Cupolas only exist with an n/d-gon as its n-face where n and d are co-prime and d is odd.  If we relax this latter condition we can consider n/d co-prime and d even. The 2n-face, being a 2n/d-gon has both 2n and d even.  This makes it a degenerate polygon which is equivalent to wrapping an n/(d/2)-gon twice around its centre.

The square and triangular faces attach to each edge of this 2n/d-gon.  As the edges of this polygon are co-incident in pairs then these edges can be removed leaving the square edge coincident with one and the triangular edge co-incident with the other.   This generates the family of semicupolas¹ (also previously known as 'cuploids').  A simple example is the 5/2-semicupola above where the pentagram is initially connected by a cupola type band of squares and triangles to a pentagon.

Bounds of 6/5 < n/d < 6 again apply, and again n and d must be co-prime, d must be even.

All of the semicupolas have the property in that if n/d > 2 then the triangular and square faces do not totally cover the 'underside' of the n-base. A small n-gonal 'membrane' is left. The 5/2-semicupola has this property and with 11 faces may be the least faced polyhedron to contain a membrane. With n/d < 2 (e.g. the 5/4 semicupola then the underside of the small base is completely covered.

This image of a 5/4-semicupola (left) and 5/2-semicupola (right) was produced by Christine Tuveson (ctuveson@earthlink.net)

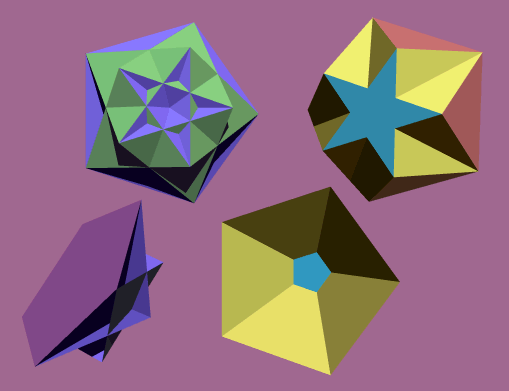
Image reproduced by permission

The 5/2 semicupola and 5/4 semicupola share faces with the ditrigonal polyhedra.  More on this relationship can be found here.
 

Any two n/d semicupolas may be joined by their n/d faces to form a toiroidal figure,  an interesting case appears to be two 5/2 cuploids being joined by their pentagrams to form the 5/2-semicupola toroid shown above.

 The 7/4-semicupola has received some specific attention.  Firstly, the beautiful image below of a 7/4 semicupola and its reflection was created by Christine Tuveson (ctuveson@earthlink.net).

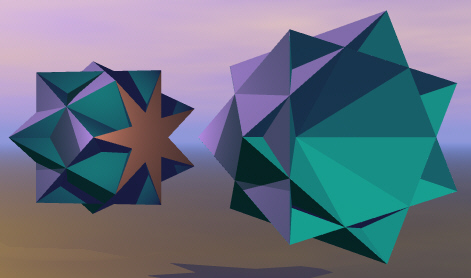
Image reproduced by permission

Secondly, the image below is of a physical model (possibly the only physical model of a cuploid in existance) created by Ulrich Mikloweit (polyart@citylink.de).  His wonderful models are unique in that he incorporates every part of each polygonal face, including the internal facets.  More images of the cuploid and of Ulrich's many other models can be found on his website at http://www.polyedergarten.de

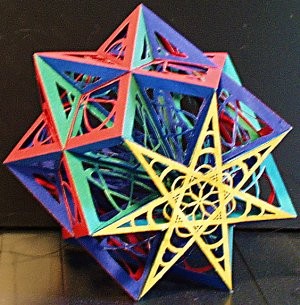
Image reproduced by permission

A second form can be generated by merging two cupolas.  Superpose both the n-face and 2n-faces.  Then rotate one cupola by 2pi/2n such that the vertices of the 2n-faces are again superposed.  The n-faces will will be gyrated with respect to each other.  These composite polyhedra is termed a sesquicupola¹ (also previously known as a  "blended gyro bicupola"). The term "blended" is required to distinguish these solids from the gyro bicupolas where the small faces are not superposed. For example, the blended 3/1 gyro bicupola is shown below, whilst the 3/1 gyro bicupola is more commonly known as the cuboctahedron.

Finally, and without any particular rationale other than forming an interesting model, for the 5/2-semicupola, it can be augmented by replacing the square faces with square pyramids to generate the strange looking almost shell like figure above.

Footnote
1. the terms 'semicupola' and 'sesquicupola' were introduced by Dr Norman Johnson in a  private communication dated December 2004.

Next: The 5/2 semicupola, the 5/4 semicupola and the ditrigonal polyhedra
Back: To Cupolas
Back: To Index