7-4-3, 9-4-3 and 11-4-3

The solutions to the 7-4-3, 9-4-3 and 11-4-3 all follow the same basic model, as does a related 5-4-3 acrohedron.  Take the base polygon, and starting with a triangle attach a ring of alternating triangles and squares around the edge, you will end with a triangle which leaves a 'V'shaped gap between itself and the first triangle (stage 1).  To the top edges of the squares attach a second ring of triangles all joined at their apices (stage 2).  The shape formed will now have a rhombus shaped opening (stage 3).  This can be shown to be planar as in effect what you have is a cupola topped by a pyramid.  The n-gonal cupola and the n-gonal pyramid have the same height.

Generate a second module identical to the first.  The two figures can be attached by their rhombus shaped openings to form a closed polyhedron.  Two orientations are possible.  Either the two base polygons can have a point in common ('ortho' mode), or the base polygons can appear at opposite sides of the rhombus ('para' mode).  A third option is to attach a 'pyramid' to the side of a 'cupola' (stage 1) and then to join two such sections with their 'cupolas' together (stage 2) to form a 'meta' mode). The constructions gives the shapes a potential name (as suggested by Alex Doskey) of 'pairwise augmented cupolas'.

5-4-3 acrohedra
ortho-decatetradihedron, 
meta-decatetradihedron,
para-decatetradihedron.

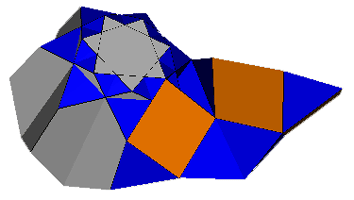
7-4-3 acrohedra
ortho-tetrakaidecahexadihedron (exploded),
meta-tetrakaidecahexadihedron (exploded),
para-tetrakaidecahexadihedron (exploded).

9-4-3 acrohedra
ortho-octakaidecaoctadihedron,
meta-octakaidecaoctadihedron,
para-octakaidecaoctadihedron.

11-4-3 acrohedra
ortho-icosikaididecadihedron (image above left),
meta-icosikaididecadihedron (image above middle) and
para-icosikaididecadihedron (image above right)

The para mode polyhedra can also exist in two isomorphous forms which are self intersecting.  Examples for the 7-4-3 are linked here:  Mode A and Mode B.

For the case of the 5-4-3 acrohedra only, it can also exist in two other alternate forms where a 'V' shaped gap from the top of the cupolaic segment is combined with a 'V' shaped gap from the side of said segment.  Models are linked here:  Mode C and Mode D

Thanks to Alex Doskey for pointing out the ortho and meta modes to me and for the alternate forms for the 5-4-3 acrohedra.

7-3-4-3 acrohedron

The 7-4-3 acrohedron and Mason Green's 7-7-3 acrohedron can be merged such that a 7-3-4-3 acron is formed.  The solution has one pair of coplanar faces which can be resolved by the excavation of a tetrahedron to give the aplanar polyhedron shown above.

Unfortunately, attempting to create a 7-4-3-3 acrohedron by rotating the 7-4-3 acrohedron results in coplanar triangles at the vertex which cannot be resolved. 

More 9-4-3 acrohedra

Using the same base elements as the 9-4-3 acrohedra above it is possible to generate further elementary polyhedra containing 9-4-3 acrons.  Rather than placing a ring of triangles and squares around an enneagon to create one rhombus (above left), it is possible to create 3 equally spaced rhombi (above right)

Three of the original modules can then be added to this base, in two different ways, to create two distinct polyhedra containing 4 enneagons as above.

Connecting two of the new '3 rhombi' modules leaves four unconnected rhombi which can again be closed by the addition of the original '1 rhombus' modules to create a polyhedron containing 6 enneagons as above

These models are to my knowledge unique in that they are (a) elemental (b) non re-entrant and (c) contain more than two apolydronic polygons.  A similar exercise for the 7-4-3 and 11-4-3 cases resulted in no solutions that were non re-entrant.  Further exploration is though possible as rhombic prisms can be inserted between the modules.

More 7-4-3 acrohedra

Further 7-4-3 acrohedra occur among the facetings of the Self Augmented Heptagonal Semi-Cupola

Back: to acrohedra