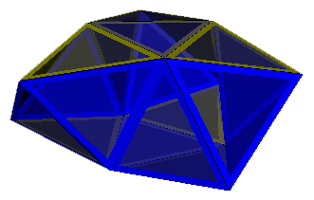
Cingulated Anti-prisms
This family of chiral prismatic polyhedra was discovered by Mason Green in 2005. It is at present the simplest such family known. It can be formed by dividing an n-gonal anti-prism into two equal sections each containing an n-gonal prismatic cap and its edge adjacent triangles, and inserting a 'cingulum' of 4n triangles. The above image shows the result for a triangular antiprism, with the cingulum shown in blue.
The family is 2-regular with vertices of (A) n/d-3-3-3-3-3 and (B) 3-3-3-3.
In the range 2 <= n/d < 2+e, the family is locally convex. e can be shown to be in the range 2/11 < e < 1/5 (or 0.1818... < e < 0.2).
For n = 2, the only convex member of the family is the Johnson Solid 'Snub Disphenoid' (J84).
Models generated to test the limit of local convexity include:
n/2 = 5/2 (2.5): Non-convex
n/2 = 7/3 (2.333...): Non-convex
n/2 = 9/4 (2.25): Non-convex. (Single loop)
n/d=11/5 (2.2): Non-convex. (Single loop)
n/d=24/11 (2.1818...): Locally-convex. (Single loop) above left
n/d=13/6 (2.1666...): Locally-convex. (Single loop) above right
The 'Single loop' entries only show one rotation around the prismatic symmetry axis and clearly show the convexity or otherwise of each example.
Examples can also be generated with n/d < 2 (e.g. this 5/3 example), no other isomorphs appear to exist.